Jinchao Xu is currently the Francis R. and Helen M. Pentz Professor of Science. His research is mainly on the design, analysis, and application of numerical methods for partial differential equations. His research interests include basic discretization schemes, grid adaptation, advanced iterative methods for solving large-scale system of equations, and applying these techniques to numerical simulations for practical problems arising for example from complex fluids, electromagnetics, material sciences, fuel cells and enhanced oil recovery.
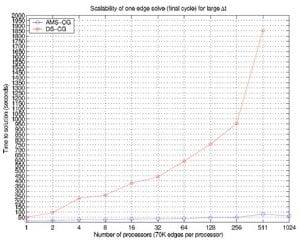
One of Xu’s main esearch interests is to develop general as well as special solvers. He developed Bramble-Pasciak-Xu preconditioner – an algorithm that is one of the two most fundamental multigrid approaches for solving large-scale discretized partial differential equations. And recently, he proposed the fast auxiliary space preconditioning (FASP) general framework, and applied to various practical problems. In 2008, the Hiptimair-Xu (HX) preconditioner, which based on FASP framework, was featured by the US Department of Energy in one of the 10 breakthroughs in computational science in recent years. Figure 1 shows the parallel scalability of the HX preconditioner in comparison with the other existing best solver.
One of the direct application of Xu’s research is the battery (including lithium ion and fuel cells) modeling and simulations. Mathematical modeling and numerical simulations are being used to currently understand the liquid water transport in the batteries, which is essential to designing the device. Xu and his collaborators developed a battery simulator, which is robust with respect to important physical and chemical parameters and converges very fast.
Xu also works on the numerical modeling of complex fluids. He and his collaborators provides a provably stable numerical scheme to simulate rheological phenomena, which leads to numerical techniques that preserve the positive definiteness of the conformation tensor for any range of Weissenberg numbers. This research represents a significant advance toward the solution of the famous High Weissenberg Number Problem.
More recently, Xu works on the numerical simulations of enhanced oil recovery. By means of FASP framework, he and his collaborators developed an efficient solver for largescale system arising from reservoir simulations, which proves to be much more efficient than commercial softwares.
